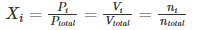What is Dalton’s Law?
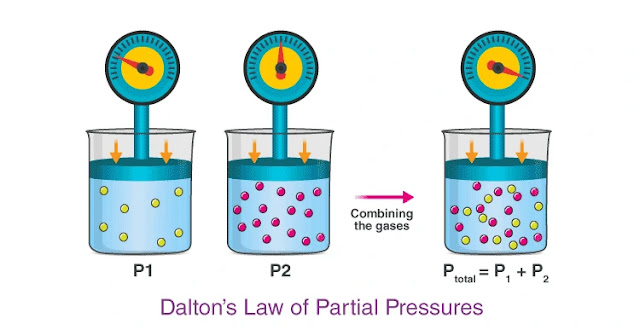
Dalton’s law of partial pressures is a gas law which states that the total pressure exerted by a mixture of gases is equal to the sum of the partial pressures exerted by each individual gas in the mixture. For example, the total pressure exerted by a mixture of two gases A and B is equal to the sum of the individual partial pressures exerted by gas A and gas B (as illustrated below).
 |
| Dalton's Law of Partial Pressures - Solved Problem & Formulas |
Dalton’s Law Formula
Dalton’s law of partial pressures can be mathematically expressed as follows:
(or) Ptotal = P1 + P2 + P3 + …. + Pn
Where,
- Ptotal is the total pressure exerted by the mixture of gases
- P1, P2,…, Pn are the partial pressures of the gases 1, 2,…, ‘n’ in the mixture of ‘n’ gases
Expressing Partial Pressures in Terms of Mole Fraction
The mole fraction of a specific gas in a mixture of gases is equal to the ratio of the partial pressure of that gas to the total pressure exerted by the gaseous mixture. This mole fraction can also be used to calculate the total number of moles of a constituent gas when the total number of moles in the mixture is known. Furthermore, the volume occupied by a specific gas in a mixture can also be calculated with this mole fraction with the help of the equation provided below.
Solved Examples on Dalton’s Law of Partial Pressure
Example 1
A mixture of hydrogen gas and oxygen gas exerts a total pressure of 1.5 atm on the walls of its container. If the partial pressure of hydrogen is 1 atm, find the mole fraction of oxygen in the mixture.
Given, Phydrogen = 1 atm, Ptotal = 1.5 atm
Applying Dalton’s law formula, Ptotal = Phydrogen + Poxygen
Therefore, Poxygen = 0.5 atm
Now, the mole fraction of oxygen, Xoxygen = (Poxygen/Ptotal) = 0.5/1.5 = 0.33
Therefore, the mole fraction of oxygen in the mixture is 0.33
Example 2
At a temperature of 300K, 30 litres of gas A kept under pressure of 1 atm and 15 litres of gas B kept under pressure of 2 atm is transferred into an empty 10L container. Calculate the total pressure inside the container and the partial pressures of gas A and gas B (Assume that A and B are ideal gases).
From the ideal gas equation, PV = nRT
Number of moles of gas A = (30L*1atm)/(0.08206 atm.L.mol-1.K-1 * 300K) = 1.22 mol
Number of moles of gas B = (15*2atm)/(0.08206 atm.L.mol-1.K-1 * 300K) = 1.22 mol
Total number of moles in the gaseous mixture = 2.44 mol
Total pressure inside the 10L container = Ptot = nRT/V
Ptot = (2.44mol*0.08206 atm.L.mol-1.K-1*300K)/10L = 6.006 atm
Therefore, the total pressure inside the 10 litre container is 6.006 atm
Mole fraction of gas A = mole fraction of gas B = (1.22 mol/2.44 mol) = 0.5
Therefore, partial pressure of gas A = Therefore, partial pressure of gas B = 0.5*6.006 = 3.003 atm
Thus, the partial pressures of gases A and B in the 10L container are both equal to 3.003 atm.