Similarity of Triangles

Triangle is a polygon which has three sides and three vertices. Triangles having same shape and size are said to be congruent. The similarity of triangles uses the concept of similar shape and finds great applications. Triangles are said to be similar if:

a. Their corresponding angles are equal.

b. Their corresponding sides are in the same ratio.

Theorems and Proofs

Theorem: If a line divides any two sides of a triangle in the same ratio, then the line is said to be parallel to the third side.

Let ABC be a triangle with sides AB, AC and BC. DE divides any two sides of the triangle in the same ratio.

Given: Line divides a triangle in the same ratio. Thus,

ADDB = AEEC

Proof:

ADDB = AEEC (Given)—-(1)

Let us assume that DE is not parallel to BC. Now we draw DE’ which is assumed to be parallel to BC. So,

ADDB = AE′E′C (Property of similar triangles)—-(2)

Therefore, from (1) and (2)

AEEC = AE′E′C

---

Now we add 1 to both sides,

AEEC + 1 = AE′E′C + 1

⇒ AEEC + ECEC = AE′E′C + E′CE′C

⇒ AE + ECEC = AE′+E′CE′C

According to the figure AE+EC = AC and AE′+E′C = AC, substituting these values in the equation above:

ACEC = ACE′C

This directly implies that EC = E′C and E = E′ meaning that they are the same point.

Hence DE is parallel to BC. This proves the similarity of triangles.

Examples

Let us take an example to observe the property of similarity of triangles:

Illustration 1:PQRS is a trapezium with PQ parallel to RS. The point X and Y are on the non-parallel sides PS and QR respectively such that XY is parallel to PQ. Show that PXXS = QYYR.

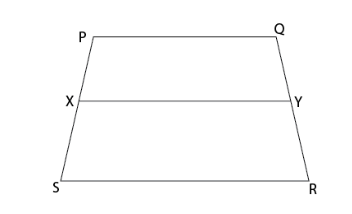
Solution: Let us first join PR in order to intersect XY at Z.

PQ || RS and XY || PQ (given)

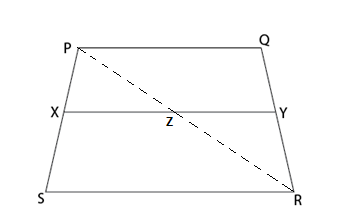
So, XY || RS (lines parallel to same line are parallel to each other)

In △ PSR,

XZ || SR (as XY || SR)

So, PXXS = PZZR …….. (3)

Similarly, from △ PRQ

RZZP = RYYQ

PZZR = QYYR …………. (4)

From equation (3) and (4),

PXXS
 = QYYR<